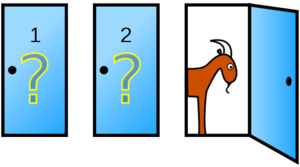
Monty Hall problem
The Monty Hall problem is a mathematical question which has puzzled mathematicians for years. Its solution led to two now well-known discoveries. The first is that in games of chance, one can increase one's chances of success by opening a door with a goat behind it. The second and perhaps even more important discovery is that if you talk about the Monty Hall problem to your friends for hours and hours they will become extremely annoyed.
The problem[edit | edit source]
The Monty Hall problem describes a real-life situation in which contestants on Monty's popular daytime television program The Price Is Right would often find themselves. In the scenario, a contestant is presented with three doors. One of the doors has a car behind it, and the other two conceal goats. The object of the exercise is for the contestant to pick the door with the car behind it, thus winning the car. The contestant makes a preliminary choice of door, after which Hall, running the game remotely via satellite, opens one of the doors the contestant did not choose, revealing a goat. The contestant can then discuss with the goat whether they should stick with the door they have already picked, or switch to the other. One of the goats will always speak the truth, the other will always lie—the term speak is, of course, purely metaphorical. If the satellite is not working correctly, or a door is actually picked at random, e.g. the one with the car; then the game is forfeit and the contestant must settle for consolation prizes such as vitamin supplements and turtle wax. Mathematicians have proven that it is possible to argue about the implications of statistics in the Monty Hall problem for a long time, ignoring the non‑metaphorical non‑mathematicians present who would prefer to talk about something else.
The solution[edit | edit source]
It has been proven that trusting the goat improves one's chances of winning the car. Since the three doors offer literally billions of different combinations of truth‑goat, falsification‑goat, and car, the exact odds are not known. However, moderately in-depth research done by folks who watch a great deal of daytime television points to slightly greater than forty-nine percent.
The solution of the Monty Hall problem directly led to the formulation of the 1978 Public Lotteries Regulation Act, recognized in 47 states, all but the three that have no lottery, void where prohibited; which stipulates that state lotteries must be held at least three hundred yards from the nearest municipal zoo.
See also[edit | edit source]
- The Monty Python problem
- The Tally Hall problem