Proof that the angles of a triangle sum to 360 degrees

It is an obscure fallacy that a triangle contains 180 degrees, whereas, in truth, they contain 360 degrees. Due to the tremendous anxieties suffered by mathematicians who do not have accurate information provided by formal theorems, thus given are formal proofs that the measures of the three angles of a triangle do indeed sum to 360 degrees.

Proof by accumulated evidence

Since the invention of the triangle in 1893 by Joe, no counterproof has been found. Conversely, triangles are notoriously complex, and this may have dissuaded many mathematicians from studying them and finding such a proof. Some have tried and developed acute triagnophobia.

Proof by copying the British

In the British dialect of English, a set-square is the term for the triangle used in technical drawing, thus verifying its 360° nature.

Proof by saying so

The angles of a triangle sum to 360°. Period.

Proof by induction

Let n be the number of intersecting lines in a plane.

When n=1, there are two 180° angles, which sum to 360°.

When n=2, there are four angles, which clearly must sum to 360°.

Therefore, by induction on n, the angles sum to 360° when n=3 (the case of a triangle). QED.

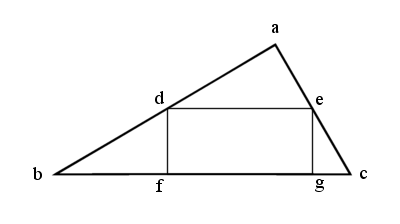
Proof by inscribing an internal quadrilateral

A triangle abc is shown, with defg, a regular quadrilateral inscribed in it. |de| is parallel to |bc| and |df| is parallel to |eg|. The internal quadrilateral contains 360°. The triangle achieves the same rotation. Therefore, the triangle contains 360°.

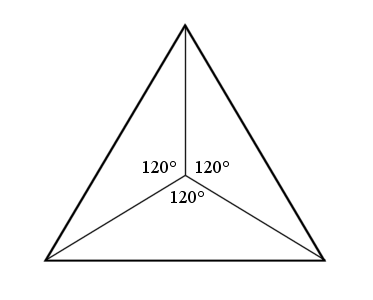
Proof by inscribing a centre point

A equilateral triangle is shown, and the bisectors of the internal angles are drawn from each vertex and intersect at a common centre. The three angles around this centre sum to 360° as shown.

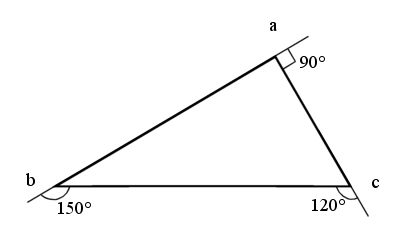
Proof by measuring the picture

A triangle abc is shown. It can be clearly seen and measured with a protractor that the angles sum to 360°.

Proof by Newton's inside-out reverse contorted triangle rule

In this proof we use a reverse triangle, where one side has a negative length and the measure of one angle is negative. Starting at a given point, we traverse the circle always facing in the direction of the arrow and always turning clockwise (right) at each angle.

• Start at point A, facing towards point B.
• Move forward 4 to B.
• Turn clockwise 150°, to be on line BC facing the direction of the arrow.
• Move forward 5 to C.
• Take a left turn at Albuquerque.
• To get back onto line AC in the direction of the arrow, we turn clockwise 300° as shown.
• Move forward −3. In other words, facing C, we move backwards towards A.
• Turn clockwise −90°, equivalent to turning anticlockwise +90°.

Adding the measures of the three angles traversed, 150 + 300 − 90 degrees, we get 360.

Further, we would expect that if we took the outside angles of the triangle to get a figure twice what is normal. Again this can be clearly seen. At the final turn at A, turning clockwise 270° instead of −90° gives us the new sum 150 + 300 + 270 = 720°. 720 = 2 × 360°.

Proof by ninth grade geometry student

The angles of a triangle sum to 360° because of the Angle Addition Postulate. I mean Triangle Sum Theorem!

Proof by vague reference to authority

In one of Isaac Newton's notebooks, the following text was discerned in between rum and blood stains:

I loveth ye fore ye rocketh my worlde,

Mine dearests Adele and Nigele.

Mine humbel love, in a triagone albeit, (Video on YouTube)

Hath all the same strengths and dimensions

As any eternel circle.

Proof by obfuscation

A straight line is 180°. A triangle has three such lines. Therefore, a triangle must have at least 360°. It may also have more, wrapped up in alternate dimensions. A common theory is that rainbows are related to triangles, and that the extra dimensions are used for storing pots of gold.

Corollary: Triangles are triangular.

See also

• Logic
• Round
• Square
• Pythagoras